Comparing Fractions Worksheet

Example 1) Compare \[ {3 \over 4} \; and \; {5 \over 6} \]

We can use diagrams to look at two fraction circles with the relevant fractions shaded.

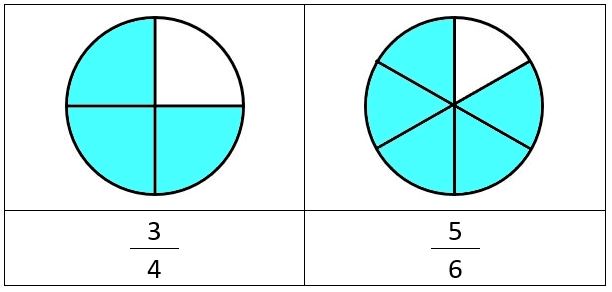
These diagrams clearly show that: \[ {3 \over 4} \] is the smaller of the two fractions as less of the diagram is shaded.

So we now know \[ {3 \over 4} \; < \; {5 \over 6} \]

Example 2) Let us compare \[ {2 \over 8} \; and \; {1 \over 4} \].

We can use diagrams to look at two fraction circles with the relevant fractions shaded.

These diagrams show the same amount shaded for each fraction, so the two fractions are equal.

We have found out that \[ {2 \over 8} \; = \; {1 \over 4} \].

Example 1) Compare \[ {1 \over 2} \; and \; {3 \over 7} \]

If we are comparing a fraction with a half, it is usually quite quick and easy to tell whether it is bigger or not.

If a fraction is equivalent to a half, then the numerator is equal to half the denominator.

In this case, half of 7 = 3.5, so if the numerator was 3.5 the two fractions would be equal, or equivalent.

However, this numerator is equal to 3, which is smaller than 3.5, so the fraction is less than a half.

So this tells us \[ {1 \over 2} \; > \; {3 \over 7} \]

Example 2) Compare \[ {2 \over 5} \; and \; {3 \over 10} \]

We cannot directly compare these two fractions until their denominators are the same!

You will notice that in this case, one of the denominators is a multiple of the other: 10 is double 5.

So all we need to do is double the numerator and denominator of the first fraction to give us an equivalent fraction with the same denominator as the second fraction.

\[ {2 \over 5} = {2 \times 2 \over 5 \times 2} = {4 \over 10} \]

We can now compare the two fractions directly by looking at the numerators as the denominators are now the same.

4 is bigger than 3 so \[ {4 \over 10} \; > \; {3 \over 10}\]

So we have found out that \[ {2 \over 5} \; > \; {3 \over 10} \]

Example 3) Compare \[ {4 \over 9} \; and \; {3 \over 5} \]

These fractions are not multiples of each other but we can see that by comparing them each to a half, one is clearly bigger and the other is smaller.

If we look at \[ {4 \over 9} \] we can see that it is less than a half because the numerator is less than half of the denominator.

If we look at \[ {3 \over 5} \] we can see that it is more than a half because the numerator is greater than half of the denominator.

This tells us that \[ {4 \over 9} \; < \; {3 \over 5} \]

Example 4) Compare \[ {3 \over 7} \; and \; {3 \over 10} \]

You will notice that these fractions do not have the same denominator but they do have the same numerator.

This really helps us to compare them, because it means that if we think of fraction diagrams the circles have been split into different numbers of parts, but both fractions have the same number shaded in.

If we consider unit fractions, where the numerator is 1.

We know that: \[ {1 \over 7} \; > \; {1 \over 10} \] because the whole has been split into fewer pieces.

This tells us that \[ {3 \over 7} \; > \; {3 \over 10} \] because we are just shading in three pieces of each circle, and each of the sevenths is bigger than each of the tenths as the diagram below shows.

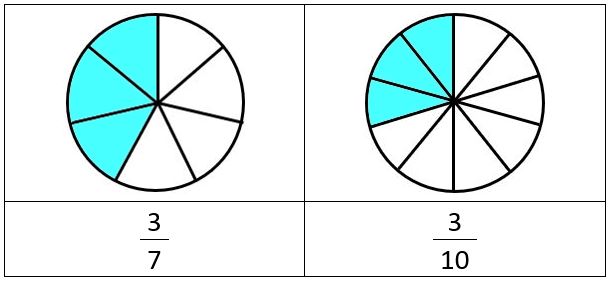
Example 5) Compare \[ {3 \over 7} \; and \; {2 \over 5} \]

If we look at both of these fractions, we can see (using the method above) that they are both smaller than a half.

We cannot directly compare these two fractions until their denominators are the same!

We now need to convert them both to fractions with the same denominator (or a common denominator) so we can compare them.

The best way to do this is to multiply the denominators together to tell us the denominator we need.

In this case 7 x 5 = 35, so we need a common denominator of 35.

To get a denominator of 35, we need to multiply the numerator and denominator of the first fraction by 5, and multiply the numerator and denominator of the 2nd fraction by 7.

This gives us: \[ {3 \over 7} \; = \; {3 \times 5 \over 7 \times 5} \; = \; {15 \over 35} \]

and \[ {2 \over 5} \; = \; {2 \times 7 \over 5 \times 7} \; = \; {14 \over 35} \]

Now that the fractions have the same denominator, we can compare the two numerators.

We can clearly see that \[ {15 \over 35} \; > \; {14 \over 35} \]

This tells us that: \[ {3 \over 7} \; > \; {2 \over 5} \]