- Home |
- About |
- Contact Us |
- Privacy |
- Newsletter |
- Shop |
- Donate
Surface Area of a Cylinder
Support Page

Welcome to our Surface Area of a Cylinder page.
We explain how to find the area of a right circular cylinder and provide worked examples and practice worksheets for you to use.
We also have a calculator will also find the surface area of a range of different types of cylinders.
Quicklinks to ...
Surface Area of a Cylinder
The surface area of a cylinder is the amount of space taken up by the two flat circular surfaces (usually the top and bottom) and the curved surface (usually the side of the cylinder).
Types of Cylinder
There are many different types of cylinders, including right cylinders, oblique cylinders and elliptic cylinders.

The right circular cylinders are the most common. They are basically a prism with two parallel circular bases directly above each other.
Oblique cylinders have circular or elliptic bases that are offset and not directly above each other.
Elliptic cylinders have two elliptic bases, instead of circular bases.
The cylinders that we use for our calculators, examples and worksheets are right circular cylinders.

Open and Closed Cylinders
As well as different types of cylinders, there are also categories of cylinder within each type: open and closed cylinders

The most common cylinder is a closed cylinder which has two parallel circular bases as well as the curved surface.
An open-top cylinder has one circular base and a curved surface but no top. A drinking glass is an example of this.
An open cylinder is just a tube which has a curved surface with no top or bottom surface.
Surface Area of a Cylinder Formulas
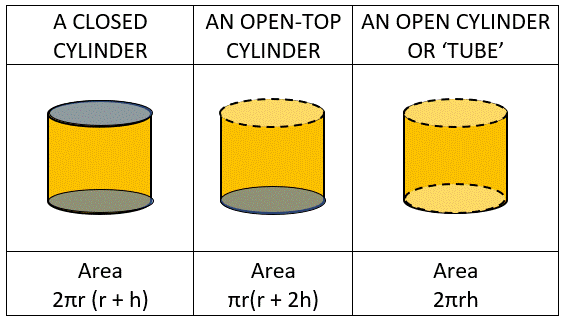
The surface area of a closed cylinder is: \[ A = 2 \pi r (r + h) \]
The surface area of an open-top cylinder is: \[ A = \pi r (r + 2h) \]
The surface area of an open cylinder (or tube) is: \[ A =2 \pi rh \]
where A is the surface area, r is the radius of the circle and h is the height of the cylinder.
How to Find the Surface Area of a Cylinder Support
If you would like to see where the formula comes from, then we hope you will find the explanation below useful.
We going to look at a closed right circular cylinder and work out the area of its net.
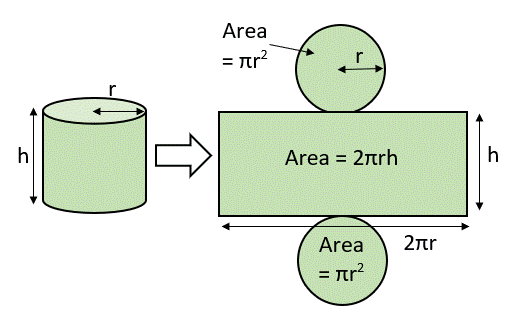
If we look at the net for the cylinder, it has two circles with radius r and one rectange with height h.
The area of a circle is: \[ A = \pi r^2 \]
The area of both circles which make up the cylinder is double this, so it is: \[ A = 2 \pi r^2 \]
The width of the rectangle is the same as the height of the cylinder, which is h.
The length of the rectangle has to be the same as the circumference of the circle as the rectangle forms a curved surface exactly around the two circular bases.
The circumference of a circle (which is also the length of the rectangle) is: \[ C = \pi d \;\ or \;\ 2 \pi r \]
The area of the rectangle is length x width \[ A = 2 \pi r \times h = 2 \pi rh \]
So if we add up the area of the 2 circles and the area of the rectangle, we get:
\[ A = 2 \pi r^2 + 2 \pi rh = 2 \pi r (r + h) \]
Area of a Cylinder Calculator
We have created a calculator to help you find the surface area of a range of open and closed right circular cylinders.
The calculator will find the area and also show you the working out step-by-step.
Surface Area of a Cylinder Examples
Area of a Cylinder Example 1
Find the surface area of the closed cylinder below to 1 decimal place.

The formula for the area of a closed cylinder is: \[ A = 2 \pi r (r + h) \]
If we substitute the values of the radius and height into this equation, we get: \[ A = 2 \pi (3) (3 + 8) = 2 \pi (3) (11) = 2 \pi (33) = 66 \pi \]
This gives us a final answer of: \[ A = 207.3 \;\ cm^2 \]
Area of a Cylinder Example 2
Find the surface area of the closed cylinder below to 1 decimal place.

The formula for the area of a closed cylinder is: \[ A = 2 \pi r (r + h) \]
As the cylinder is lying on its side, the actual 'height' of the cylinder is 6ft and the radius is 1 ½ ft
If we substitute the values of the radius and height into this equation, we get: \[ A = 2 \pi (1 {1 \over 2}) (1 {1 \over 2} + 6) = 2 \pi (1 {1 \over 2}) (7 {1 \over 2}) = 2 \pi ({45 \over 4}) = {45 \over 2} \pi \]
This gives us a final answer of: \[ A = 70.7 \;\ ft^2 \]
Area of a Cylinder Example 3
Find the surface area of the closed cylinder below to 2 decimal places.

The formula for the area of a closed cylinder is: \[ A = 2 \pi r (r + h) \]
We have been given the diameter instead of the radius. To find the radius, we just need to halve the diameter.
So the radius of the circular base is: \[ Radius = 4.8 \div 2 = 2.4 m \]
If we substitute the values of the radius and height into this equation, we get: \[ A = 2 \pi (2.4) (2.4 + 1.7) = 2 \pi (2.4) (4.1) = 2 \pi (9.84) = 19.68 \pi \]
This gives us a final answer of: \[ A = 61.83 \;\ m^2 \]
Area of an Open-Top Cylinder Example 4
Find the surface area of the drinking glass shown below. Give your answer to 1 decimal place.

The glass is an open-top cylinder.
The formula for the area of a open-top cylinder is: \[ A = \pi r (r + 2h) \]
We have been given the diameter instead of the radius. To find the radius, we just need to halve the diameter.
So the radius of the circular base is: \[ Radius = 7 \div 2 = {7 \over 2} cm \]
If we substitute the values of the radius and height into this equation, we get: \[ A = \pi ({7 \over 2}) ({7 \over 2} + 2 \times 16) = \pi ({7 \over 2}) ({71 \over 2}) = \pi ({497 \over 4}) = {497 \over 4} \pi \]
This gives us a final answer of: \[ A = 390.3 \;\ cm^2 \]
Area of a Tube Example 5
Find the surface area of the relay baton shown below. Give your answer to 1 decimal place.

The relay baton is a hollow tube.
The formula for the surface area of a tube is: \[ A =2 \pi r h \]
We have been given the diameter instead of the radius. To find the radius, we just need to halve the diameter.
So the radius of the circular base is: \[ Radius = 1 {1 \over 2} \div 2 = {3 \over 4} inches \]
If we substitute the values of the radius and height into this equation, we get: \[ A = 2 \pi ({3 \over 4}) (12) =2 \pi (9) = 18 \pi \]
This gives us a final answer of: \[ A = 56.5 \;\ in^2 \]
Surface Area of a Cylinder Worksheets
We have 3 different worksheets for you to practice the skill of finding the surface area of a range of cylinders.
The first sheet involves finding the surface area of different closed cylinders when the radius and height are known.
The 2nd sheet involves finding the surface area of different closed cylinders when the diameter and height are known.
The 3rd sheet involves finding the area of a range of closed, open-top and open cylinders when the diameter and height are known.
More Recommended Math Worksheets
Take a look at some more of our worksheets similar to these.
Volume of a Cylinder
More Surface Area Worksheets and Support Pages
More Circle Worksheets and Support Pages
More Area Support and Resources
We have a range of other area worksheets and support pages for a range of different 2d shapes.
Looking for more math calculators?
We have a wide range of free math calculators to help you.
Most of our calculators show you their working out so that you can see exactly what they have done to get the answer.
Our calculator hub page contains links to all of our calculators!
How to Print or Save these sheets 🖶
Need help with printing or saving?
Follow these 3 steps to get your worksheets printed perfectly!
How to Print or Save these sheets 🖶
Need help with printing or saving?
Follow these 3 steps to get your worksheets printed perfectly!
Subscribe to Math Salamanders News
Sign up for our newsletter and get free math support delivered to your inbox each month. Free seasonal math grab pack included.
Math-Salamanders.com

The Math Salamanders hope you enjoy using these free printable Math worksheets and all our other Math games and resources.
We welcome any comments about our site or worksheets on the Facebook comments box at the bottom of every page.




















New! Comments
Have your say about the Math resources on this page! Leave me a comment in the box below.